如何彻底删除C盘下的windows.old文件夹
本文共 292 字,大约阅读时间需要 1 分钟。
本步骤不用任何第三方工具,即可将顽固的windows.old文件夹删除,操作有风险,诸君需谨慎!!!
- windows + R 键 打开运行窗口 输入
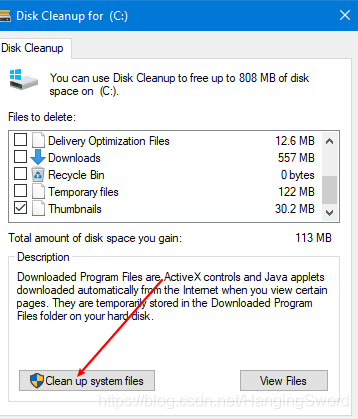
cleanmgr,出现下面的窗口后直接点击确定即可
- 接着点击下图中的“清理系统文件”,过一会儿会出现跟下图中差不多的选项
 3. 在要删除的列表中找到上个版本的Windows安装,向上滑动还有一个Windows更新的日志文件,这个可以选择删除,根据自己的需求勾选想要删除的内容后点击确定即可。
3. 在要删除的列表中找到上个版本的Windows安装,向上滑动还有一个Windows更新的日志文件,这个可以选择删除,根据自己的需求勾选想要删除的内容后点击确定即可。 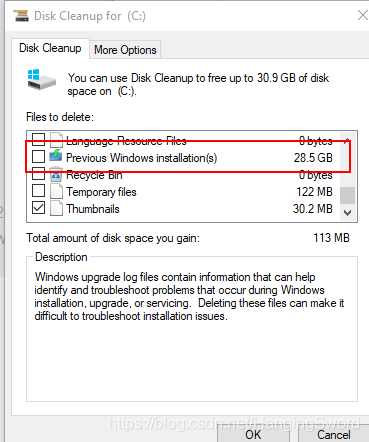 4. 等一会儿会出现一个警告,问你要不要继续,点击确定即可。
4. 等一会儿会出现一个警告,问你要不要继续,点击确定即可。
提示:将windows.old删除后,会存在无法还原到上个版本Windows的风险
转载地址:http://pmkj.baihongyu.com/
你可能感兴趣的文章
MySQL杂谈
查看>>
mysql权限
查看>>
mysql条件查询
查看>>
MySQL架构与SQL的执行流程_1
查看>>
MySQL架构与SQL的执行流程_2
查看>>
MySQL架构介绍
查看>>
MySQL架构优化
查看>>
MySQL查看数据库相关信息
查看>>
MySQL查看表结构和表中数据
查看>>
MySQL查询优化:LIMIT 1避免全表扫描
查看>>
MySQL查询优化之索引
查看>>
mysql查询储存过程,函数,触发过程
查看>>
mysql查询总成绩的前3名学生信息
查看>>
MySQL查询报错ERROR:No query specified
查看>>
mysql查询数据库储存数据的占用容量大小
查看>>
MySQL查询数据库所有表名及其注释
查看>>
MySQL查询数据表中数据记录(包括多表查询)
查看>>
MYSQL查询语句优化
查看>>
mysql查询语句能否让一个字段不显示出来_天天写order by,你知道Mysql底层执行原理吗?
查看>>
MySQL死锁套路:一次诡异的批量插入死锁问题分析
查看>>